
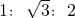
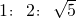
 应用题天天练四川大学出版社系列答案
应用题天天练四川大学出版社系列答案科目:初中数学 来源: 题型:
查看答案和解析>>
科目:初中数学 来源: 题型:
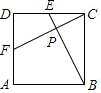 Suppose that in Fig.2,the length of side of square ABCD is 1,E and F are mid-points of CD and AD respectively,GE and CF intersect at a point P.Then the length of line segment CP is
Suppose that in Fig.2,the length of side of square ABCD is 1,E and F are mid-points of CD and AD respectively,GE and CF intersect at a point P.Then the length of line segment CP is查看答案和解析>>
科目:初中数学 来源: 题型:
查看答案和解析>>
科目:初中数学 来源:竞赛题 题型:单选题
A. 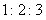
B. 
C. 
D. 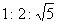
查看答案和解析>>
湖北省互联网违法和不良信息举报平台 | 网上有害信息举报专区 | 电信诈骗举报专区 | 涉历史虚无主义有害信息举报专区 | 涉企侵权举报专区
违法和不良信息举报电话:027-86699610 举报邮箱:58377363@163.com